Solving Equations: Variables on Both Sides vs Combine Like Terms

It seems pretty cut and dry to me - either the variables are on the same side of the equal sign or they aren't. But I can't tell you how many of my students confuse the procedures for these equations. I find that showing one of each of these types of problems side by side helps them. 

So I would show these two equations and ask them to compare and contrast them. I also have them draw a line down the equal sign when learning how to solve equations. This really helps them to remember to keep both sides balanced using the properties of equality. 

I created these notes to help walk students through the difference between the two types of equations. I like to use gradual release with these notes. We solve the equations at the top together (I Do). Then they try the matching in the middle with a partner (We Do). Then they try to problems at the bottom on their own (You Do). I usually project these notes onto the board and have students show their work on the board. 

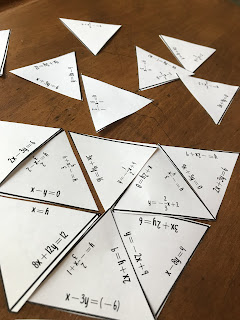
Like any other math topic, practice helps them to improve so I created this puzzle . I love using self-checking activities so students can self- monitor as they go. If they don’t find their answer or the puzzle doesn’t make the correct shape, they know they made a mistake. I tell them the first thing they should check is whether they correctly combined like terms or used inverse operations.  Often they will incorrectly use inverse operations when solving a problem where the terms are on the same side. They will say “I subtracted 7x from both sides.” Then it’s very easy to have them show me where they did that on the other side (spoiler alert - they didn’t) and then we can talk about a different strategy instead.

Graphing Equations from Standard Form

My students have such a difficult time keeping track of all the different methods of graphing lines from each form. I use this foldable to help them keep track of the various forms, but I also drill that they can always change it to slope-intercept form. And if they get really desperate, they can just use a table of values to graph everything. I think practicing converting from standard form to slope intercept form is a great way to practice literal equations as well.

I use these notes to practice rearranging equations from standard form to slope-intercept form. I have students glue them along the edge of the page and show all their work on the lines of the notebook paper. (They print four per page.)

I created this puzzle for students to practice converting between standard form and slope-intercept form. They find an equation in standard form, rearrange it to slope intercept form, and match the two pieces. There are several similar equations to keep students on their toes and "attending to mathematical precision."

 I love using these puzzles for practice activities, not only does it encourage students to work together, but students also receive immediate feedback - when they don't find their answers or their puzzle doesn't make the right shape, they know they have a mistake.

Translating a line

I will never forget one of my students telling me after a district exam, "One of the questions asked me to translate a line - what does that mean, write it in Spanish?" I thought I had done just a great job on the linear functions unit, but I had totally missed connecting the idea of translations for them. I had always waited until quadratic functions to talk about shifts in the parent function as transformations, but when I looked into the standards I realized I was missing a key part of linear functions. CCSS.MATH.CONTENT.HSF.BF.B.3: Identify the effect on the graph of replacing f(x) with f(x)+ k, … for specific values of k (both positive and negative); find the value of k given the graphs. 

So I created some doodle notes that compare various graphs to the parent function. Each graph already had y = x graphed, so seeing the transformation is easier. 

The standard specifically addresses translations, but I also wanted students to see that changing the slope is actually a dilation and making it negative is a reflection. 

After finishing the notes, we completed a dominoes activity to practice translations. I love these dominoes because they have an answer bank to work from. They start with the line y = x and perform the vertical translations up or down. Students match the graph and then perform the next translation.

For extra practice I had them write the equation of each line, again reinforcing the idea that the constant is the only thing changing in the equation. 

Both sheets made up a two-page spread in our interactive notebooks. 

I wrapped things up with this "Try It" question too. I know that before this lesson, my students would have had no idea how to even tackle it, but they were so confident in their answers. With any luck, when they see questions about "translating" on the next test, it will no longer think of a foreign language.